📌 Harmonics and Heterodyning of Frequencies

[MUSICMUSICMUSIC]

Harmonics and Heterodyning of Frequencies

A perfectly symmetrical square wave produces major odd harmonics, that is, AC frequencies at 1,3,5,7,9… times its frequency. The power available in those harmonics decreases as the multiplication factor increases. For example, a 100Hz signal produces harmonics (AC frequencies) at 100, 300, 500, 700, 900, … but at strengths of 1, 1/3, 1/5, 1/7, 1/9 … of the original. These harmonics extend to the radio frequency range and beyond, to infinity, theoretically.

Frequencies also exhibit a heterodyning effect. This is explained by an excerpt from a web site:

From http://www.taralabs.com/

---

[…] consider a sound wave of 264 cycles (middle C on the Scale of Just Intonation). In order for this tone to be perceived as both accurate and natural, one must be able to hear not just the 264 cycle tone itself, but the higher order harmonics which extend well up into the frequency range vulnerable to RF modulation.

This intermodulation creates an effect called heterodyning, where additive and subtractive tones, known as beat frequencies, are created. For example, mixing two frequencies of 100 KHz and 1 KHz results in the following frequencies being created:

100 KHz

1 KHz

101 KHz

99 KHz

These frequencies will also combine to produce beat frequencies of their own. Add in a multiplicity of RF signals and it is easy to imagine the degree to which RF alters and distorts the musical signal. The effect is especially evident in the higher frequency bands.

---

Since square waves are used, this is not just mixing two frequencies. It is mixing all the odd harmonics. Instead of producing 100 and 1, we are producing 100, 300, 500, 700, and all the additions and subtractions.

Now, combine the effects of harmonics with heterodyning with this example:

Consider a 10Hz square wave. It is producing a strong third harmonic at 30Hz (at 2/3 less power). Heterodyning means it is also producing 30 - 10 = 20Hz and 30 + 10 = 40Hz. The 5th harmonic is 50. 50-10 = 40Hz. This means that 40 gets a “boost” since it is produced in these two ways, among others. While this process goes up the scale with infinitely more numbers it produces a huge range of frequencies of varying intensities (all at less than the power at the base frequency.

[MUSICMUSICMUSIC]

Harmonic Visualization Models – Sacred Geometry

The study of sacred geometry was passed down over thousands of years from the ancient mystery schools. The most common geometries considered sacred are the Egyptian Flower of Life, the Hebrew Vesica Piscis and the Italian Borromean Rings though there are others.

Harmonic Sacred Geometry

The reason such geometry was considered sacred is due to their similarity to organic geometries found in life. Such geometry was described as ’emanations’ by Gnostics, indicating their awareness that they were patterns formed by the intersection or interference patterns of waves. And since music travels in waves, sacred geometry became intertwined with musica universalis, the ancient theory of everything. Through musical proportions, everything could be modeled using sacred geometry.

To see how this works, consider the following musical Chromatic Ring divided into twelve equal sections according to the earlier harmonic color model. This is known as the Z/12Z cyclic ring model where Z is the set of integers 1 through 12.

Harmonic Sacred Geometry 2

The labels indicate bi-lateral symmetry between each half of the ring as divided by the Harmonic Axis through the Harmonic Center or harmonic Partial 9. Spring symbols are used to indicate the regions where harmonics share energy, namely between the tritone and tonic major third.

From the Chromatic Ring, a dual ring system can be used to geometrically represent the standard Fourier wave consisting of complementary wave components sine and cosine in phase-quadrature (out of phase by 90-degrees).

Harmonic Sacred Geometry 3

To the ancients, this interlocking ring arrangement was known as the Vescia Piscis or Mandorla (meaning ‘almond’), referring to the union section of the two rings. In the Hebrew Kabbalah, all life is described as entering into the world through the mandorla interference region between the dual rings.

In the most general sense, this is correct. The dual rings are a vertical cross-section of the double torus produced by the Coriolis effect – a geometry found in the formation of galaxies and our own solar system. Life too is toroidal like a hurricane, twisting around an axis, as seen in the swirl of hair at the crown of the head. When we are born, the birth canal is indeed a mandorla.

Harmonic Sacred Geometry 4For these and other reasons, the mandorla is used to frame religion icons and deities in Christianity, indicating their origination from the creation matrix. Moving beyond religious interpretations, we can better understand the physical properties of the dual rings by separating the twelve tones into two groups of six divided between the rings – one group in the top ring to represent sine components in phase with the Harmonic Center (D for harmonic series of C) and another group in the bottom ring to represent the complementary cosine components. The two groups of tones are known as whole tone scales, of which there are only these two.

Interestingly, we find that the mandorla corresponds to the so-called ‘dominant’ interval (e.g., {G, B} in C major), used in common practice music to create tension and resolve to the tonic. This so-called ‘dominant-tonic’ resolution is also used as a central element in music harmony to chain together a series of chords into what is called the Cycle of Fifths. When represented as a lattice of interlocking Vesica Piscis, dominant triads can be seen to cascade through an orbit of twelve tones.

Harmonic Sacred Geometry 5

When this same lattice progression is then transformed onto an orthogonal wave lattice using dominant 7th chords, something truly amazing happens. The Cycle of Fifths traces out the profile of a fish. In fact, the tense leading tone adds onto itself in such a way as to produce a prominent fin-like geometry – appearing very shark-like. Sacred geometry indeed!

Harmonic Sacred Geometry 6

Not only is life is born through a mandorla, but harmony of form is the inevitable self-organization process of nature. As harmonic waves reflect inside round containers, they assume the same proportional shapes found in Z/12Z music harmony – a bi-lateral symmetry of harmonic proportion. Sacred geometry is nothing less than the visualization of physical musical concepts.

[MUSICMUSICMUSIC]

What is Resonance?

Example: Acoustical resonance of different frequencies in a cello.

1. A phenomenon of reflected energy
2. Always occurs inside some kind of container
3. Has a prime resonant frequency
4. Represented as squared magnitudes (E=mc2)
5. Responsible for all coherence in nature
6. Universal to ALL types of waves (air, water, electricity, light, atoms, plasma, etc.)

Neural Resonance

• Holonomic brain theory (Karl Pribram, David Bohm)

‘Cognitive function is guided by a matrix of neurological wave interference patterns.’

• Harmonic resonance theory (Steven Lehar)

‘Spatial patterns in perception and behavior are mediated by spatial standing waves in neural tissue.’

What is a Standing Wave?

As waves reflect in a container, the prime resonant frequency reflects back on itself (adding and subtracting in opposition) to create a stationary oscillating wave pattern. Similarly, all interference patterns are composed of standing waves that are similarly stable and stationary as well.

As a standing wave forms, additional waves can form in the same medium. These waves are called ‘harmonics,’ ‘wave partials’ or in music ‘overtones.’ Harmonic partials to the standing wave are not random frequencies, but instead whole number multiples (1x, 2x, 3x, … nx) of the prime resonant frequency. This is called the harmonic series .

This is what the harmonic series looks like when both opposing phases of interference are superimposed at a constant energy level or amplitude. Notice the stable pattern it creates and the gaps that form around the nodes and other locations. This is universal to all kinds of waves as they ‘resonate’ inside some container.

Question is, what causes standing waves to form in such a regular pattern? What is the underlying physics that prevents fractional or enharmonic waves from forming on the prime resonant frequency?

[MUSICMUSICMUSIC]

What is Resonance?

Example: Acoustical resonance of different frequencies in a cello.

1. A phenomenon of reflected energy
2. Always occurs inside some kind of container
3. Has a prime resonant frequency
4. Represented as squared magnitudes (E=mc2)
5. Responsible for all coherence in nature
6. Universal to ALL types of waves (air, water, electricity, light, atoms, plasma, etc.)

Neural Resonance

• Holonomic brain theory (Karl Pribram, David Bohm)

‘Cognitive function is guided by a matrix of neurological wave interference patterns.’

• Harmonic resonance theory (Steven Lehar)

‘Spatial patterns in perception and behavior are mediated by spatial standing waves in neural tissue.’

What is a Standing Wave?

As waves reflect in a container, the prime resonant frequency reflects back on itself (adding and subtracting in opposition) to create a stationary oscillating wave pattern. Similarly, all interference patterns are composed of standing waves that are similarly stable and stationary as well.

As a standing wave forms, additional waves can form in the same medium. These waves are called ‘harmonics,’ ‘wave partials’ or in music ‘overtones.’ Harmonic partials to the standing wave are not random frequencies, but instead whole number multiples (1x, 2x, 3x, … nx) of the prime resonant frequency. This is called the harmonic series .

This is what the harmonic series looks like when both opposing phases of interference are superimposed at a constant energy level or amplitude. Notice the stable pattern it creates and the gaps that form around the nodes and other locations. This is universal to all kinds of waves as they ‘resonate’ inside some container.

Question is, what causes standing waves to form in such a regular pattern? What is the underlying physics that prevents fractional or enharmonic waves from forming on the prime resonant frequency?

[MUSICMUSICMUSIC]

How do harmonics form?

As waves reflect and resonate inside a container or cavity, they cross one another. As they cross, they exchange energy at specific locations called ‘damping wells.’ In quantum mechanics this is explained by Landau-Zener theory (1932).

Known as Landau damping , waves that pass through one another mostly transparently, avoiding a direct collision, are called ‘avoided crossings.’ In such cases, energy is exchanged in a ‘parameter zone’ where one wave pushes against another, creating a kind of spinning well or vortex action. Like a kind of switch, energy is passed ‘adiabatically’ (without heat loss) across the damping well in a kind of torque action.

We can think of the damping well as a kind of low-pressure zone much like those in our atmosphere that create storms, hurricanes and tornados. The surrounding pressure differential causes an implosion toward the center of the low-pressure zone, forming a vortex.

In the special case of a standing wave, damping wells form at constant locations at golden sections of the period of the prime resonant frequency. This is because the golden ratio constant, represented by the Greek letter _ (or Phi) and equal to the ratio of about 1 : 0.618033… or 1.618033, is non-reflective while having the unique ability to nest into itself infinitely. As a result, energy is exchanged between harmonics at Phi proportions while harmonic wave partials begin to ripple outward from the edge of the well.

Because of this, the damping well of a standing waves can be described as a Fibonacci spiral converging to Phi, the deadest location in a standing wave and thus the point of greatest torque and energy exchange between harmonics. This can be proven by using the Fibonacci series as a nominal solution for the second-order equation known as the ‘characteristic wave damping equation.’ In this proof, the golden ratio Phi becomes the ‘eigenvector’ and the Fibonacci series (1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, …} become its ‘eigenvalues.’

In fact, we can understand harmonic formation as something called ‘phi-heterodyning’ where each harmonic emerges around the Phi eigenvector in nested golden sections like a fractal. A paper by Bovenkamp and Giandinoto entitled ‘Incorporation of the Golden Ratio Phi into the Schrödinger Wave Function using the Phi Recursive Heterodyning Set’ shows how this can occur, but there is an easier way to visualize it.

The Fibonacci damping well approximates the irrational Phi Recursive Heterodyning Set with rational harmonic waves. Above the proportion of 13:8, wave formation is damped and suppressed while below this in the range of {1 … 12} whole number harmonics can form and resonate constructively with the prime resonant frequency. In general, waves that come too close to Phi proportions in the resonant frequency are killed while those furthest away resonate the most.

While this sounds like a new idea, it is actually part of the age-old practice of creating resonant chambers that suppress the formation of unwanted standing waves and reflection. Stradivarius was well-known for using golden sections in the design of his violins. The best rectangular concert halls use golden proportions in their dimensions, as do modern day speaker enclosures.

Standing wave reflection simply cannot be sustained when one of the three dimensions in a container is at or even near a golden ratio to another.

[MUSICMUSICMUSIC]

Harmonic patterns in nature

The first step in understanding harmonic resonance patterns is to study cymatic patterns created by vibrating sand or water.

Each pattern is the result of specific harmonic frequencies reflecting inside a container, such as a circular cup of water. For instance, a pentagonal geometry is formed either by one frequency resonating at the 4th wave partial of the prime resonance frequency (4 : 1 = 5 vertices) or two frequencies forming an interval, such as a perfect fifth (3 : 2 = 5 vertices). In both cases, we simply add the two sides of the harmonic proportion to find the shape of geometry.

Cymatic patterns can also form in low-pressure zones that form between two regions of pressure differential (described in an earlier discussion about damping wells).

In a spinning bucket of water, cymatic patterns arise from the velocity differential between the outer edge of the bucket and its center axis. As before, the geometry is determined by the frequency proportion created.

Swirling weather patterns work the same way, spiraling around a center while closing into an approximated circle in the center. In recent years, the most powerful storms occasionally form cymatic patterns in the clouds, even tracing out a pentagram inside a pentagonal eye. Similar formations have been found on other planets, such as a giant hexagon around Saturn’s south pole.

While it is obvious that the bucket acts as a container for the standing wave patterns in water, what can we say is the container for weather patterns? Most would answer ‘clouds’ or ‘the planet’ but the more correct answer is gravity. Gravity acts as a damping well in space around a planet, forming a spherical bubble within which waves can reflect and form stable patterns.

The same thing happened long ago in the plasma disc of our solar system, forming planets in orbits spaced approximately at right angles along a Golden Spiral. In the process, the planets and their moons exhibit a variety of resonant properties. The most obvious is the resonant orbit of Venus to Earth.

In the heliospheric current sheet of the sun, a Phi-damping well once existed in the plasma between Earth and Venus. At one edge of the well was the planet node Venus and at the other Earth. For every 13 orbits that Venus travels around the Sun, Earth travels almost exactly 8, creating the resonant proportion 13 : 8 (mentioned in the harmonic formation section). Since 13 / 8 = 1.625, the two orbits straddle the stabilizing Phi-damping eigenvector in the space between, forming the geometry of a pentagonal star in the two planet’s conjunctions.

This star cymatic pattern was well known in ancient times as the Star in the East, the Morning Star, the Babylonian Star and the Star of Bethlehem. Since it rose in the sky before the Sun, it was called the ‘light bringer’ or in Latin ‘Lucifer.’ This orbital star pattern played a prominent role in many early religions and associated with the sacred feminine presence of God. This included the Hindu Vena (and Shukra), Kali, Ishtar, Innana, Astarte, Semiramis, Ashtoreth, Isis, Aphrodite, Venus, Demeter, Hathor, Ostara and Eastre.

But, celestial mechanics are not the only place where harmonics help nature organize itself. At the atomic level, harmonic resonance between atoms is known as bonding. In the case of life, 99 percent of a living thing is made of water and carbon and the way in which these atomic geometries harmonize. In particular, both assume pentagonal connectivity maps at the mesoscopic level where molecules cluster together.

As carbon-water clusters fit together to form cells, organs and bodies, life assumes a range of organic shapes. Nature’s geometry is not strictly a matter of natural selection and random mutation. Atomic resonance is also at work, guiding the formation of different life forms into a limited number of possibilities which are then differentiated further by environment.

For humans, the pentagonal shape was the guiding harmonic pattern, creating a dual-pentagon arrangement in the DNA molecule, a pentagonal cranium and five fingers and toes on each side of our body. In this way, physiological shape can be described as a harmonic patterning process that emerges from a Fibonacci spiral – exactly like that of a harmonic standing wave heterodyning out of a golden ratio fractal.

Note that the golden ratio Phi = (1 + √5) / 2

[MUSICMUSICMUSIC]

Cymatics Today, a Window into the Universe
The underlying principle of cymatics is that the geometry of sound can be imprinted onto membranes and made visible with special techniques. The membrane can be a flexible material, such as latex or your skin, while other surfaces, such as brass or glass plates, may appear rigid yet they can still be minutely imprinted by sound. Simply by sprinkling on a little powder or sand, provided the membrane is horizontal, the imprint of sound can be revealed. The particulate matter gathers in the areas that are not vibrating, leaving the vibrating areas clear of particulate. Cymatic patterns are, therefore, rather like a photographic negative, because they represent the inverse of the sound that caused them to form.

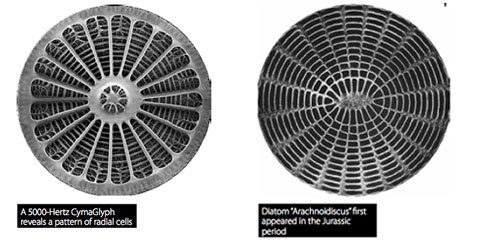
Below is a typical CymaGlyph created with sand on the CymaScope that reveals a fundamental frequency of 5000 Hertz. Note the similarity between this cymatic pattern with the structure of a diatom, a sea creature that first appeared in the oceans of the Jurassic period, around 185 million years ago.

We used sand in our early research as the disclosing medium, but we soon discovered that water, with its highly flexible surface tension, acts like a super-thin membrane and reacts almost instantly to any sound, revealing very high levels of detail.

In the water CymaGlyph below an ultra- pure tone of 22.2 Hertz demonstrates archetypal pentagonal geometry creating an almost 3D view of sound. This CymaGlyph compares remarkably with the structure of the Campanula flower.

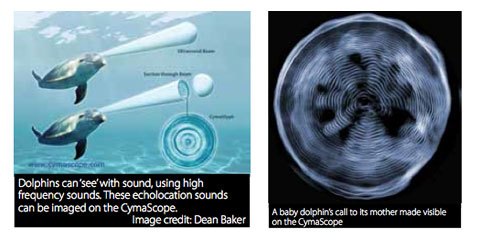
Dolphin Research
Applications for the CymaScope are beginning to emerge in many different fields. For example, in collaboration with Jack Kassewitz of SpeakDolphin.com we have taken the first steps in unraveling the mystery of dolphin language. We are contributing to their research program by transcribing dolphin echolocation sounds into CymaGlyphs, with each image representing a type of dolphin picture word. The image below is that of a CymaGlyph created by a baby dolphin calling to its mother. The call creates a replicable pattern of acoustic energy with a particular meaning. The second graphic illustrates the basic principle: a cross section through the dolphin’s high frequency sound beam is made visible on the CymaScope.

Signature Sounds of Stars

Another application for the CymaScope is imaging sounds from space. We discussed earlier the concept that all sounds have an infrared component. When we speak or sing outdoors our words or song will one day reach the stars in the form of modulated infrared light. But the reverse is also true: sounds from stars continually bathe the earth. Oscillating stars have a particular type of signature and in collaboration with Professor Don Kurtz we recently imaged the sound of a star that he discovered, known as HR 3831-A. This technique allows us to see the distinctive geometry of the sounds at work within the atomic furnace of the star and could provide a valuable analogue for future students of asteroseismology and for outreach projects in schools and colleges.

Cymatics in Egypt
Most people who have experienced the acoustics of the King’s Chamber in the Great Pyramid walk away with a feeling of awe, in some cases coupled with an impression that the chamber was designed to reverberate. For a relatively small chamber the reverberation is indeed extraordinary; one can literally hear one’s own breathing (when the fluorescent lighting is turned off) and this experience often accompanies feelings of cathedral-like reverence. This notion of design implies a prior knowledge of acoustics and materials. The high levels of reverberation in the chamber are actually a function of the flat granite surfaces, their parallel arrangement and the chamber’s dimensions.

John Stuart Reid undertook an experiment in the Great Pyramid in order to investigate his belief that the King’s Chamber was designed to be highly reverberant and that the energy of any sound made in the chamber is transferred into the sarcophagus. Reid has studied Egyptology for decades and believes that the King’s chamber was designed to support a rebirthing ritual enacted prior to the pharaoh’s death or perhaps afterwards and that vowel sounds chanted in the chamber were intended to have an energizing effect on the sarcophagus and its occupant during sacred rituals.

Cymatics Experiment in the King’s Chamber
Reid conducted his cymatics experiment in 1997 with the intent of making sounds within the sarcophagus visible. He positioned a speaker inside the sarcophagus and connected it to an oscillator, a device that electronically creates pure tones. Finally, he sprinkled quartz sand on the surface area of the temporary latex membrane stretched across the sarcophagus and turned the oscillator on. To Reid’s amazement as well as the ‘Keeper of the Keys’ who accompanied him in the chamber, an astounding array of ancient Egyptian hieroglyphic-like images appeared! Two are shown here and we have many others on file.

The simplest explanation for how hieroglyphs came to be imbedded in the sarcophagus derives from the highly resonant crystalline structures imbedded in the granite that would have bathed the scribes in sound bubbles during the construction of the sarcophagus. Hypothetically, one or more of the scribes had synaesthetic abilities, that is, they could ‘see’ the sounds from the sarcophagus while it was being worked with tools. Reid postulates that these minds-eye sound patterns influenced the scribes in the development of the hieroglyphic language.
(For those readers interested to learn more about Reid’s acoustics research in Egypt please go to our web site: http:// http://www.cymascope.com/cyma_research/ egyptology.html)

Cymatics and the Chakras
The word ‘chakra’ means wheel or circle in Sanskrit and is based on the ancient Eastern model of the body having seven primary chakras or energy centres. Each of the seven centres connects subtle energies with the physical body and they are receptors that vitalize the body with prana or life force energy. The chakras are aligned from the base of the spine to the top of the head. Each chakra possesses specific purposes and is associated with the functioning of a particular endocrine gland.

In order to maintain emotional, mental, physical and spiritual health, all chakras must be functioning properly and working harmoniously with each other. When one or more of the chakras become imbalanced or blocked the result is dissonance in certain aspects of the body. Emotional imbalance, illness or disease often manifest as a result. Just as the cells of our bodies produce distinct sounds, so too do the chakras, although science is only just beginning to investigate this phenomenon using SQUID magnetometers.

Many healers have used their intuition to guide them to the sounds of the chakras and one of the most notable authorities on this subject is Jonathan Goldman, renowned sound healer. He devised a system of chakra sounds that Reid imaged on the CymaScope and can be used as an aid in meditation. Astoundingly, when he imaged the heart chakra it appeared in the shape of a heart—the image below has not been retouched except for the addition of colour.

The study of cymatics is still in its infancy and innumerable realms within this sphere of research are waiting to be explored. Please visit our website for updates on the latest cymatic insights and discoveries.

[MUSICMUSICMUSIC]

Seeing Sound with the CymaScope
Sound is an invisible force that permeates every aspect of our lives. With the exception of music, many man- made sounds are jarring while the sounds of Nature tend to flow over and around us like soothing waters, lifting our spirit, inspiring us, exciting us. Yet if we could see sound our world would be even more beautiful than we could imagine. It would be a world filled with shimmering holographic bubbles, each displaying a kaleidoscopic pattern on its surface. To see sound is to open a new window onto our world, one that has been veiled in mystery until recently.

When the microscope and telescope were invented centuries ago, new realms came into view that were not even suspected to exist—a Universe in miniature under the microscope and a Universe so immense that centuries of research lie before us with the telescope. Now, like the microscope and telescope that preceded it, the CymaScope instrument allows us to see a previously invisible realm—the world of sound— helping us to gain a deeper and fuller understanding of life and the Universe. The CymaScope uses the science of ‘cymatics’ to make sound visible, by imprinting sound’s invisible vibrations onto the surface of ultra pure water to reveal its once-hidden geometric structures.

This new scientific frontier reveals aspects of Nature every bit as authentic as a flower or a butterfly, the stars in the heavens or starfish in the oceans—in fact, as we will come to see in this article, sound is just as much at work in the interior of a star as iis in the organs of a starfish or in the cells of your body. Sound lies at the heart of every aspect of Nature, underpinning all of Creation. Cymatics will, in the future, enable humanity to understand far more about the Universe and our world than was possible with previous technologies. The CymaScope and the science of cymatics provide a bridge that will lead to significant advancements in knowledge.

The Shape of Sound
Before looking at cymatics more closely let us dispel the popularly held misconception that ‘sound is a wave’. It isn’t. All audible sounds are, in fact, spherical in form or spheroidal , that is to say audible sounds are sphere-like but not necessarily perfectly spherical. For the sake of simplicity we’ll call these spheroidal sound spheres ‘sound bubbles.’

Our world is teeming with beautiful holographic sound bubbles that envelop us in shimmering patterns of acoustic energy, each bubble rushing away at around 700 miles an hour as new bubbles form from the source of the sound. Whether the sound is emitted from your voice or from some other source, such as a musical instrument, this ‘bubble-in-a-hurry’ leaves a fleeting vibrational imprint on the surface of your body: every cell in the surface tissues of your body actually receives sound patterns from the bubbles that surround you. However, only low frequency sounds can penetrate the interior of your body. To understand more fully how your cells respond to the healing power of audible sounds please refer to our previous Veritas article, Rediscovering The Art And Science Of Sound Healing .

Yet, despite the fact that sound is not a wave, the term ‘sound wave’ is in general use throughout the world, which is rather amazing when sound waves don’t actually exist! So let us briefly discuss how this strange anomaly has occurred.

Sound is basically periodic movements of air molecules bumping into each other. These movements of sound can be described mathematically and when plotted graphically the shape of the graph does indeed look like a wave. However, if we could see audible sounds shimmering in the air around us we would see beautiful bubbles, not waves, so it is misleading to say that sound is a ‘wave.’ If what is actually a bubble is described as a wave it is possible that incorrect conclusions will be made about the way Nature works.

In the illustration below a slice through a sound bubble is depicted. The peaks of the graph represent the regions of high-pressure air within the sound bubble, whereas the mid points of the graph represent the areas of low-pressure air. The ‘space-form’ of audible sound is indeed bubble-like whereas the graph—often referred to as a sound wave—is merely a mathematical depiction of the peaks and valleys of sound pressure.

Sound and its Relationship with Light
To understand the concept of visual sound a little more fully it will be helpful to explore how the vibrating atoms of air that create sound relate to light and life. At the moment of these atomic sound collisions something quite magical happens: Light is created.

Light occurs every time the magnetic shells of two vibrating atoms bump against each other. The frequency of light created in this way depends on the energy in the collisions, meaning how fast they bump together. Try this experiment: Rub your hands vigorously together. You’ll feel warmth. This is because the atoms in one hand are slipping past the atoms in your other hand, creating heat, which is just another name for light. The light you create by this friction method is in the infrared part of the spectrum of electromagnetism, invisible to our eyes but quite visible to some species of bat, owl, snake and mosquito.

You create infrared light even when you speak. The atoms and molecules in the air are excited by the vocal folds in your larynx, creating a tiny pearl of acoustic energy that rapidly expands out of your mouth and rushes away at around 700 miles an hour. The atoms and molecules of air within this expanding bubble are bumping into each other, each collision transferring your voice vibrations to the nearest atom or molecule. As these ‘bumps’ occur they cause infrared light to be created due to the friction between the magnetic shells of the air particles. The infrared light carries with it the modulations of your voice that rush away at the incredible speed of 186,000 miles per second. Unlike the sound of a voice, which becomes inaudible after about one mile, the infrared light created by your voice rushes out into space where it travels for eternity, carrying your words or songs to the stars.

Thus, there is a direct relationship between sound and light and in fact there can be no light in the Universe without sound because light is only created when atoms collide with each other, and such collisions are sound. So light and life owe their existence to sound.

[MUSICMUSICMUSIC]

Thanks